5 Things to Know for the ACT Mathematics Section

Think of this page as your emergency ACT Math study lifeline. 🛟 Math can be rough and sometimes it feels like you don't even know what you don't know. We will take you through 5 general areas that will give you a solid foundation for what you need to know when you sit down to crunch those ACT numbers. 

Below is a short outline of the five areas of your ACT math lifeline. 

1. • ACT Math Section Structure — The Basics
2. • ACT Math Topics and Formulas to Know — Just what is on this darn test?
3. • ACT Math Toolbox — “Who” can help me? (Aka, what tools can I use?)
4. • ACT Math Section Tips and Tricks — Cheatcodes for this section
5. • ACT General Tips and Tricks — Cheatcodes for the ACT in general

---

🏗️ 1. ACT Math Section Structure

Well, of course, the first thing you must know in order to succeed in the math section of the ACT is its structure! This will give you a basic idea of how you should pace yourself during the test, what topics you need to review, and other information that you can use to help you do well on the test. We will go into this further later in the guide. Here is a breakdown of the ACT math section:

• • There are 60 questions to be answered in 60 minutes.⏲️
• • All are five-choice, multiple-choice questions.📄
• • There is no formula sheet.😭(Don't fear though! We'll go over the most important ones in the next section.)
• • You are allowed to have a test-approved calculator for the entire section.🧮
• • You will receive 9 scores for this section—1 score for the section overall, and 8 (sub)category scores which are calculated based on specific mathematical knowledge and skills. We have listed them below, and we will talk about each of them more in-depth in the next section:- - Preparing for Higher Math (57–60% of questions)- - Number and Quantity (7–10%)- - Algebra (12–15%)- - Functions (12–15%)- - Geometry (12–15%)- - Statistics & Probability (8–12%)- - Integrating Essential Skills (40–43%)- - Modeling

---

🧪 2. ACT Math Topics and Formulas to Know

Now that we have been introduced to the very basics of the ACT math section, let’s dive a little deeper—is knowing how to play Geometry Dash enough?? ⏹️ (Spoiler: The answer is NO!) 

In this section, we’ll go over what exactly you need to know, including commonly tested formulas. 🧪 

Remember, the ACT does NOT provide a formula sheet!

Preparing for Higher Math (57–60% of the Math section)

Number and Quantity (7–10% of the questions on the test are classified as this type of question)

According to the ACT, this subcategory asks students to 

“Demonstrate knowledge of real and complex number systems. Reason with numerical quantities in many forms, including expressions with integer and rational exponents and vectors and matrices.” Here are the most important formulas you need to know for this subcategory:

Logarithms

• • Rewriting logarithms:- - log_a(b) = c → a^c = b- - log_a(bc) = log_a(b) + log_a(c)- - log_a(b/c) = log_a(b) − log_a(c) Rates
• • quantity = rate * time- - distance = speed * time Sequences
• • Arithmetic sequence: a_n = a_1 + d(n-1)- - a_n = nth term of sequence- - a_1 = first term of sequence- - d = the common difference between two adjacent terms- - example: 1, 5, 9, 13, … where d = 4
• • Geometric sequence: a_n = r^(n-1)a_1- - a_n = nth term of sequence- - a_1 = first term of sequence- - r = the common ratio between two adjacent terms- - example: 2, 8, 32, 128, … where r = 4

Algebra (12–15%)

According to the ACT, this subcategory asks students to

“Solve, graph, and model multiple types of expressions. Interpret and use many different kinds of equations, such as linear, polynomial, radical, and exponential relationships. Find solutions to systems of equations, even when represented by a simple matrix equation, and apply results to real-world contexts.” Here are some formulas you need to know for this subcategory:

Linear relationships 

• • Slope-intercept form: y = mx + b- - m = slope = (y_2 – y_1) / (x_2 – x_1)- - b = y-intercept = (0, b)
• • Distance formula: sqrt[(y_2 – y_1)^2 + (x_2 – x_1)^2]- - Derived from the Pythagorean Theorem! 📐
• • Midpoint formula: midpoint = [(x_1 + x_2)/2, (y_1 + y_2)/2] Quadratic relationships
• • FOIL (First, Outer, Inner, Last): (a + b)(c + d) = ac + ad + bc + bd
• • Quadratic formula: x = (-b ± sqrt(b^2 - 4ac))/2a- - Discriminant = b^2 - 4ac- - Discriminant > 0 → 2 distinct real solutions- - Discriminant = 0 → 1 distinct real solution- - Discriminant < 0 → 0 real solutions

Functions (12–15%)

According to the ACT, this subcategory asks students to

“Demonstrate knowledge of function: definition, notation, representation, and application. Use functions including linear, radical, piecewise, polynomial, exponential, and logarithmic. Manipulate and translate functions, as well as interpret and use important features of graphs.” Here are some concepts you need to know for this subcategory:

Function notation

• • f(x) = a function named f- - x represents the input value or independent variable- - f(x) represents the output value or dependent variable
• • f ∘ g(x) = f(g(x))

Geometry (12–15%)

According to the ACT, this subcategory asks students to

“Apply your knowledge of shapes and solids, using concepts such as congruence and similarity relationships or surface area and volume measurements. Apply your understanding to composite objects and solve for missing values in triangles, circles, and other figures. Use trigonometric ratios and equations of conic sections.” Here are the formulas you need to know for this subcategory:

Lines and angles

• • Complementary angles add up to 90 degrees
• • Supplemetary angles add up to 180 degrees

The “C” in complementary stands for “Corner” like a right angle. The “S” in supplementary stands for “Straight” like a line.

• • Vertical angles are congruent

Image Courtesy of CueMath

Triangles

• • Angles of a triangle add up to 180 degrees
• • Area of a triangle = 1/2 * base * height
• • Pythagorean theorem: a^2 + b^2 = c^2- - a, b = legs of triangle- - c = hypotenuse of triangle

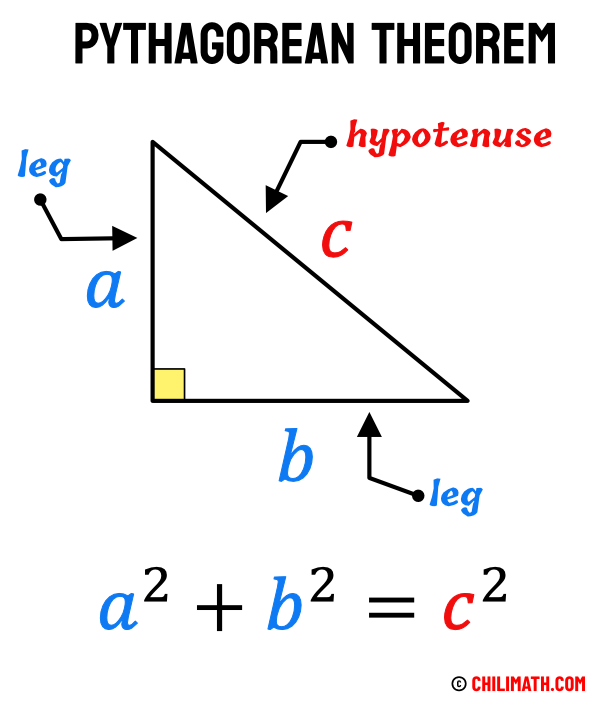
Image Courtesy of ChilliMath

• • Ratio between the sides of a 45-45-90 triangle: 1:1:sqrt(2)
• • Ratio between the sides of a 30-60-90 triangle: 1:sqrt(3):2 Polygons
• • Perimeter of n-sided polygon = side_1 + side_2 + side_3 + side_4 + … + side_n = sum of all side lengths
• • Areas of polygons- - Area of a rectangle = length * width- - Area of a parallelogram = base * height- - Area of a trapezoid = (base_1 + base_2)/2 * height

The area of a n-sided polygon (n > 4) can usually be found by adding the areas of the lesser-sided polygons that compose the polygon

• • Angles of a n-sided figure add up to (n - 2) * 180 degrees 3D figures
• • Rectangular prisms- - Volume of a rectangular prism = length * width * height- - Surface area of a rectangular prism = 2(length * width + length * height + width * height)
• • Right cylinders- - Volume of right cylinder = π * radius^2 * height Circles
• • Diameter of a circle = 2 * radius
• • Circumference of a circle = 2π * radius
• • Area of a circle = π * radius^2
• • Arc length of a circle = (central angle / 360 degrees) * circumference
• • Sector area of a circle = (central angle / 360 degrees) * area
• • Standard equation of a circle: (x – h)^2 + (y – k)^2 = r^2- - Center of a circle = (h, k) Parabolas
• • Vertex form: y = a(x – h)^2 + k- - a, h, k are constants- - Vertex = (h, k)- - Axis of symmetry: x = h Trigonometry
• • Soh-cah-toa- - sin(x) = opposite/hypotenuse- - cos(x) = adjacent/hypotenuse- - tan(x) = opposite/adjacent
• • Trig identities- - tan(x) = sin(x)/cos(x)- - sin(x) = cos(90 degrees - x)- - cos(x) = sin(90 degrees - x)- - sin^2(x) + cos^(x) = 1

Statistics & Probability (8–12%)

According to the ACT, this subcategory asks students to

“Describe center and spread of distributions. Apply and analyze data collection methods. Understand and model relationships in bivariate data. Calculate probabilities by recognizing the related sample spaces.” Here are some concepts you need to know for this subcategory:

Percents

• • % = divide by 100
• • Percent of b that is a = a/b * 100%
• • a% of b = a/100 * b Statistics
• • Mean (average) = sum of terms/number of terms
• • Median = middle number in a sorted list
• • Mode = most frequent number in a list
• • Range = largest number - smallest number Probability
• • Probability of 1 = event is guaranteed to happen
• • Probability of 0 = event will never happen
• • Probability of an event = P(event) = number of outcomes in desired event / total number of possible outcomes
• • P(event) + P(event doesn’t happen) = 1
• • P(event A and event B) = P(event A) * P(event B) Counting
• • Number of combinations = total number of possible outcomes for an event = (num of outcomes for element 1) * (num of outcomes for element 2) * (num of outcomes for element 3) * … * (num of outcomes for element n)- - n = number of elements part of event

Integrating Essential Skills (40–43% of the Math Section)

According to the ACT, this category asks students to

“synthesize and apply understandings and skills to solve more complex problems.” You will be asked to solve problems that involve multiple steps and applications in real-world contexts. 

Here are some concepts that fall under this category:

• • rates and percentages
• • proportional relationships
• • area, surface area, and volume
• • average and median
• • expressing numbers in different ways Having a thorough understanding of what the formulas from “Preparing for Higher Math” mean will help you score well in this category!

Modeling

According to the ACT, this category asks students to

"produce, interpret, understand, evaluate, and improve models. Each question is also counted in other appropriate reporting mathematics categories. This category is an overall measure of how well you use modeling skills across mathematical topics."

See additional information on the above topics in the Fiveable ACT Math Study Guides

• • ACT Math: Preparing for Higher Math: Number and Quantity
• • ACT Math: Preparing for Higher Math: Algebra
• • ACT Math: Preparing for Higher Math: Statistics & Probability
• • ACT Math: Essential Skills: Data Analysis & Representations
• • ACT Math: Integrating Essential Skills: Modeling

---

🧰 3. ACT Math Toolbox

On test day, it will be just you and your brain 🧠, but there are some tools⚙️that can aid you!

✏️__Paper + Pencil!__

• • This one may seem “silly” but writing and sketching things down on your test booklet will actually be very beneficial.
• • While the work you show on your test won't be graded, writing solutions down will make it easier for you to pinpoint mistakes and fix them.🔨
• • Geometry questions are often difficult to answer without looking at a diagram. However, they will not always be provided, so create one yoursel using your sketching skills!🖌️ 📲 Your (graphing) calculator!!!
• • The ACT allows you to have your calculator for the entire duration of the math section, so use it!
• • You should use your calculator for complex equations with big numbers as it will save you a ton of time.⌛However, for easy operations, you should avoid using it or be really careful.

Always make sure you enter in the correct numbers and operators! Especially negatives ➖

• • If you have a graphing calculator 📈, utilize it for questions asking what the graph of a function looks like or what function a graph shows. Make sure to familiarize yourself with how to do this on your specific calculator.

---

🪄4. ACT Math Section Tips and Tricks

When taking tests, it is normal to feel overwhelmed and stressed out. Here are some tips and tricks💡that will help you feel more confident and increase your score!

❓🔚 Finish reading the ENTIRE question before you start working on it. Make sure you answer what the question is actually asking.

• • This might seem like common sense, but following this tip can help you stop making simple mistakes and increase your score!
• • For example, let’s look at the following problem: When x = 3 and y = 5, by how much does the value of 3x^2 – 2y exceed the value of 2x^2 – 3y?

F. 4

G. 14

H. 16

I. 20

J. 50

Credits: ACT, Inc — Question 2 from The ACT Test Math Practice Test Questions

If a student doesn’t carefully read the entire question, they might just plug in x = 3 and y = 5 to 3x^2 – 2y and call it a day. However, the question asks how much 3x^2 – 2y is greater than 2x^2 – 3y by. Reading all the way will help one from committing a “careless error.”

🔢🔌Plug numbers in!! This strategy is one of the most useful for the math section of the ACT (or other standardized tests). It has 2 applications, which we will go over below.

• • Application 1: Pick easy numbers to work with and plug them into the question’s variables. Then, check the result against the answer choices!- - This application is good for questions asking you to find an equivalent expression.
• • Application 2: Plug the numbers from the answer choices into the variables in the question and see which choice yields the right result!- - This application is good for questions asking you to find the solution to an equation or system of equations.

BONUS: Combine this tip with ✏️ Paper + Pencil! to sketch graphs of functions

---

✨ 5. ACT General Tips and Tricks

Here are some more tips and tricks💡that you can apply to any section of the ACT (or even any standardized test for that matter)!

🦘 If you get stuck on a question, DON’T PANIC! Don’t continue to spend time on it either. Skip it, solve as many other questions as you can, and return to it if you still have time.

• • Every question on the ACT is weighted equally. So, if you waste your time trying to solve a hard question, you might run out of time to solve easier questions.

Make sure to always put down a random guess since there's a 20% chance that you will get it right.

🔁 P-R-A-C-T-I-C-E! (Yes, practice makes (more) perfect.) Just reading about concepts and tips and tricks won’t actually improve your score. You have to practice applying them! You should get to the level where you can tell what a problem is testing and how to approach it as soon as you finish reading it. 

When you answer questions incorrectly on practice tests, try to figure out why you got it wrong and why the correct answer is correct.

---

💫 Conclusion

As you continue your ACT Math journey, keep these five things in mind to help you succeed. Believe in yourself! You got this! ✨