7.1 Gravitational Forces

Overview

Gravitation may be the last and shortest unit, but you can see the applications of everything you've learned so far in this unit! From momentum to energy to forces, everything has accumulated to this point.

Big Ideas

• Fields:- How does the moon stay in orbit despite its great distance from the Earth?
• Conservation:- Why is navigation technology dependent on the orbits of Earth’s artificial satellites?

Exam Impact

Unit 7 will cover approximately 6%-14% of the exam and should take around 5 to 10, 45-minute class periods to cover. The AP Classroom personal progress check has 10 multiple choice questions and 1 free response question for you to practice on.

Newton's Law of Universal Gravitation and Gravitational Forces 🍎

When an object is large enough/massive enough it will create its own gravitational field which can interact with other objects that have gravitational fields.

** Newton's Law of Universal Gravitation: Every object attracts every other object in the universe with a force that is directly proportional to the product of their masses and inversely proportional to the square of the distance/radius between their centers.**

Newton's law of universal gravitation is a fundamental physical law that describes the attractive force between two masses. The law states that every point mass in the universe attracts every other point mass with a force that is proportional to the product of their masses and inversely proportional to the square of the distance between them. Here are some key points about Newton's law of universal gravitation:

• The force of attraction between two masses is given by the equation F = G * (m1 * m2)/r^2 where F is the force, G is the gravitational constant, m1 and m2 are the masses, and r is the distance between the centers of the masses.
• The gravitational constant (G) is a fundamental constant of nature and has a value of 6.67 x 10^-11 N*m^2/kg^2
• The force of gravity is always attractive, and acts along the line connecting the two masses
• The gravitational force between two masses is independent of the nature of the masses and depends only on the product of their masses and the distance between them.
• The gravitational force between two masses decreases with the square of the distance between them, making it weaker as the distance increases.
• The law of universal gravitation is a long-range force, meaning that it acts over large distances, and it is the force responsible for the orbits of planets, the motion of galaxies, and the overall structure of the universe.
• It is important to note that the law of universal gravitation was the first physical law to explain the motion of celestial bodies with mathematical precision. It was developed by Sir Isaac Newton and was included in his famous work "Philosophiæ Naturalis Principia Mathematica" (Mathematical Principles of Natural Philosophy) published in 1687.

You can see the equation form of the law below:

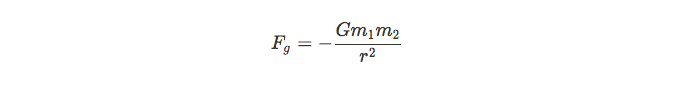
In which Fg is the force of gravity, m1 and m2 are masses, r is the distance between the two masses, and G is the universal gravitational constant which is 6.6710^(-11) ((m^3)(kg*s^2)).

Essentially, this law is an extension of Newton's Third Law in which every action has an equal and opposite reaction.

Here's a diagram that illustrates the law:

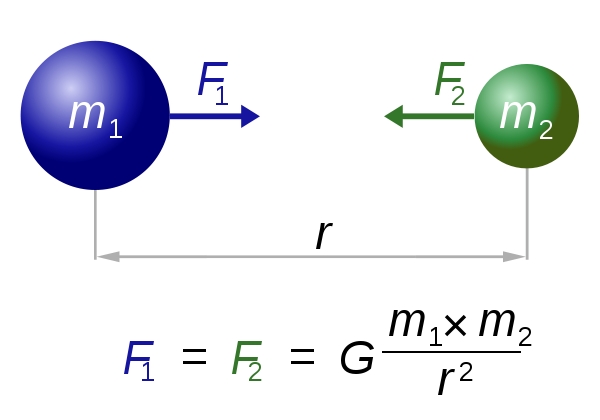
Taken from Wikimedia Commons 

Now, we can use this equation to find the acceleration due to gravity on an object!

If the object is on/near earth's surface:

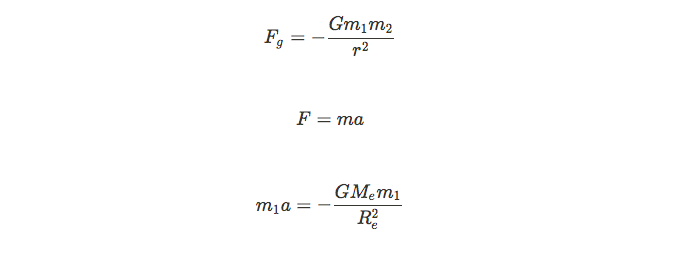
g is the acceleration due to gravity and we can cancel out the mass, therefore:

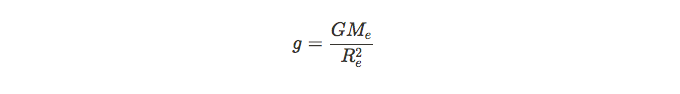
if the object is far from the earth's surface, we use the distance from the earth to the object rather than the earth's radius:

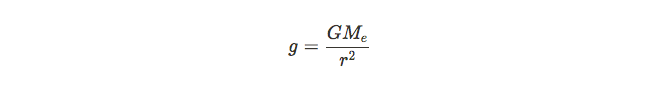
Additionally, it should be noted that gravity is a conservative force, meaning that its path is independent of it and the total work on a closed path is zero. Note that work done by a conservative force is equal to the negative change in potential energy. However, it is not uniform! 

This might be intuitive to some, but the further away the object is, the weaker the gravitational force acting on it from the other object will be. You can see this below:

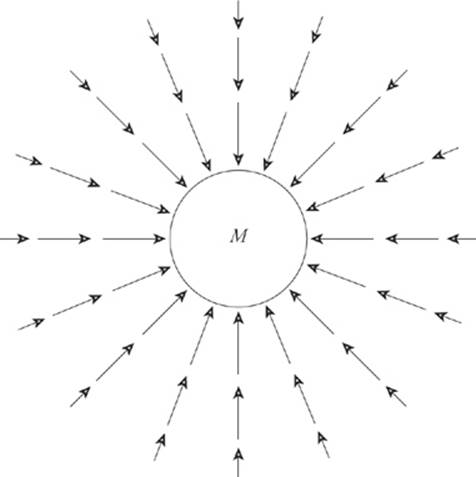
Taken from Physics Stack Exchange 

Motion of an object under the influence of a variable gravitational force

When an object is dropped from a great height and falls towards the Earth's surface, the force of gravity acting on the object is variable. As the object falls, it accelerates due to the increasing force of gravity acting on it.

At the beginning of the fall, the object will have a relatively slow velocity, and its acceleration will be small. As the object falls further, its velocity increases, and its acceleration also increases. At some point, the object will reach its terminal velocity, which is the maximum velocity it can reach due to the resistance of the air.

As the object continues to fall, its velocity will remain constant and its acceleration will be zero. As the object approaches the Earth's surface, the force of gravity will decrease, and the object's velocity will decrease as well.

Finally, the object will hit the Earth's surface, and its motion will come to an end. The impact will be the result of the force of gravity acting on the object throughout its fall, and it will be affected by the height from which the object was dropped, and the resistance of the air.

Overall, the motion of an object falling towards the Earth's surface from a great height can be described as an acceleration followed by a constant velocity, then a deceleration before the impact.

Unit 7.1 Practice Problems

Taken from College Board 

Answer

Answer