<< Hide Menu
3.7 Sinusoidal Function Context and Data Modeling
3 min read•june 18, 2024
Kashvi Panjolia
Kashvi Panjolia
Equation of a Sinusoidal Function
In this guide, you will learn how to construct the equation of a sinusoidal function from a graph. There are a few steps involved in the process, and this topic will give you an even deeper understanding of the equation we saw in the previous guide. To construct the equation of a sinusoidal function from a graph of the function, we can use the properties of the function to determine the parameters in the equation.
The general form of the equation of a sinusoidal function is:
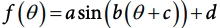
or
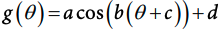
Where:
- a is the amplitude, which is half the distance between the maximum and minimum values of the function.
- b is the coefficient that determines the period and frequency of the function.
- c is the horizontal shift, which determines the position of the function along the x-axis.
- d is the vertical shift, which determines the position of the function along the y-axis.
Constructing the Equation
Consider the graph below. Let's try to find the sinusoidal equation for this function.
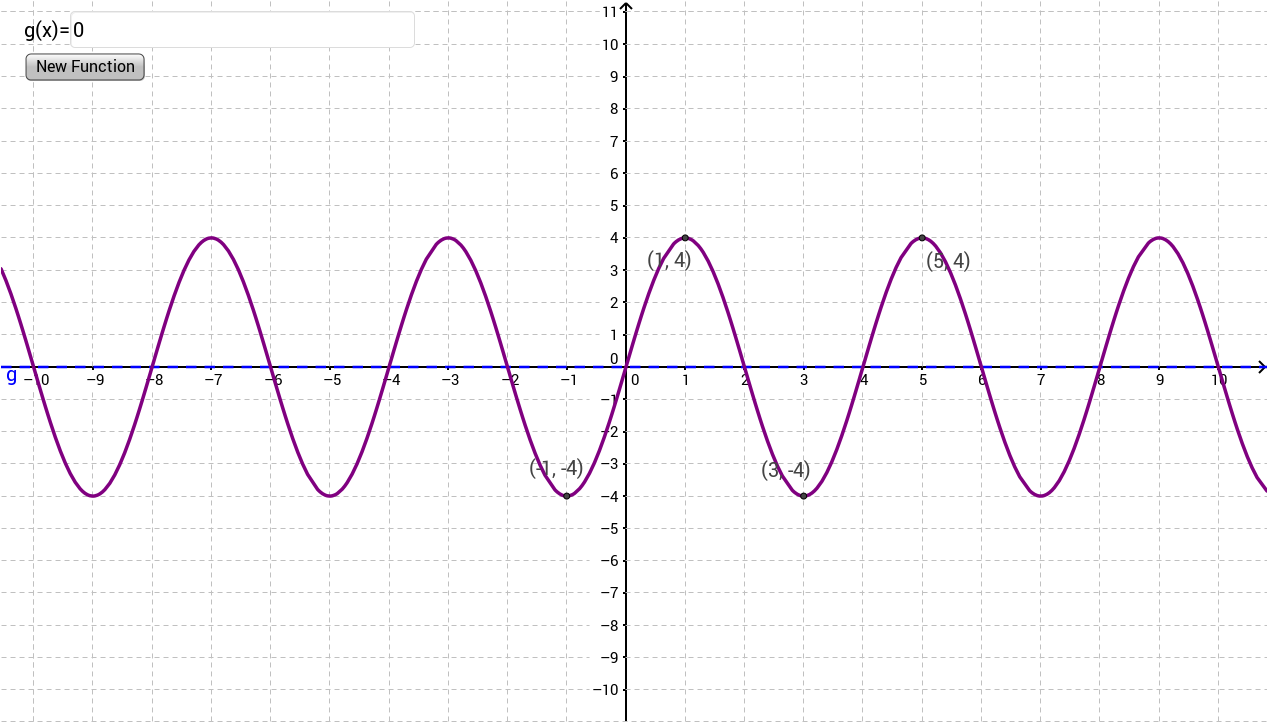
Image courtesy of GeoGebra.
To determine the amplitude of the function, we can measure the distance between the maximum and minimum y-values on the graph and divide by 2. The minimum y-value is -4, and the maximum y-value is 4. Adding together the absolute values of these numbers and dividing by two, we get an amplitude of 4.
To determine the period and frequency of the function, we can look at the x-values of the points where the function repeats its maximum or minimum values. The period of the function is the distance between these x-values, and the frequency is the reciprocal of the period. For the graph above, the function peaks at x=1, and the next peak is at x=5. Therefore, the period of the function is 5-1=4. The frequency, the value that goes into the equation, is the inverse of the period. However, for sinusoidal functions, since the period of the sine function is 2𝛑, the frequency of a sinusoidal function can be calculated by 2𝛑/period. In this case, the frequency would be 2𝛑/4, or 𝛑/2.
To determine the vertical shift of the function, we can look at the position of the function along the x- and y-axis. The vertical shift is the value of the shift of the midline of the function. The original sine function has a midline at y=0, so by finding the midline of the graph, you will be able to find the vertical shift. The midline can be calculated by adding together the maximum and minimum y-values and dividing by 2, but without taking their absolute value. For this graph, the midline is calculated using [4 + (-4)]/2 = 0, so the midline is at y=0 and the vertical shift is 0. Notice how we used the negative in the value of the minimum of the graph, unlike before when we were calculating amplitude.
To determine the phase shift of the functions, you need to find the other values of the function first. Since we have determined these values, we can use a point on the function and our equation to find the phase shift (horizontal shift). We can substitute the values we found into the equation, and our point, to solve for the phase shift. Picking the point, (1, 4), we can solve for the phase shift like this:
f(θ) = 4sin((𝛑/2)(θ + c)
4 = 4sin((𝛑/2)(1 + c)
c = 0
Our phase shift is 0. Remember that the argument of the sine function (what goes inside the parentheses) is in radians, not degrees.
Once we have determined the values of these parameters, we can substitute them into the general form of the equation to get the equation of the sinusoidal function.
amplitude = (4 + |-4|) / 2 = 4
period = 4
frequency = 2𝛑/period = 2𝛑/4 = 𝛑/2
horizontal (phase) shift = 0
vertical shift = 0
f(θ) = asin(b(θ + c)) + d
f(θ) = 4sin((𝛑/2)(θ + 0)) + 0
f(θ) = 4sin((𝛑/2)θ)
This is the equation of the sinusoidal function represented by the given graph.
Practice Problems
Construct the equations of the graphs below.
1.
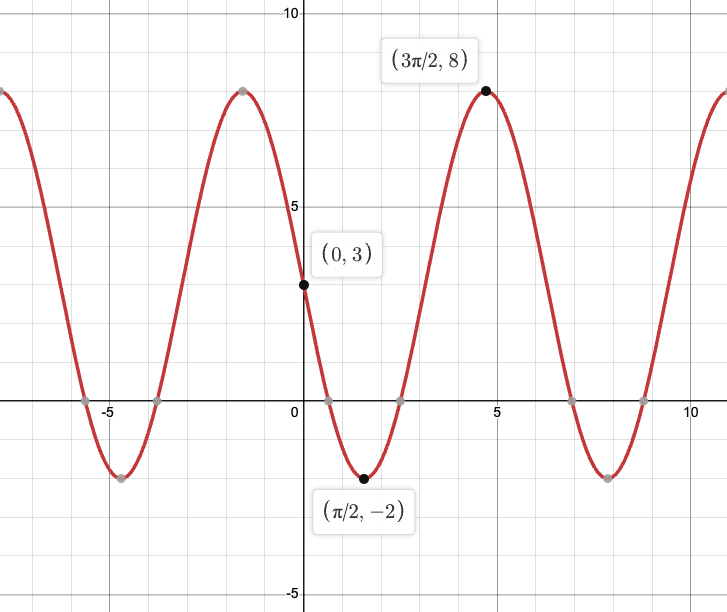
Answer: f(x) = 5sin(x + 𝛑) + 3
2.
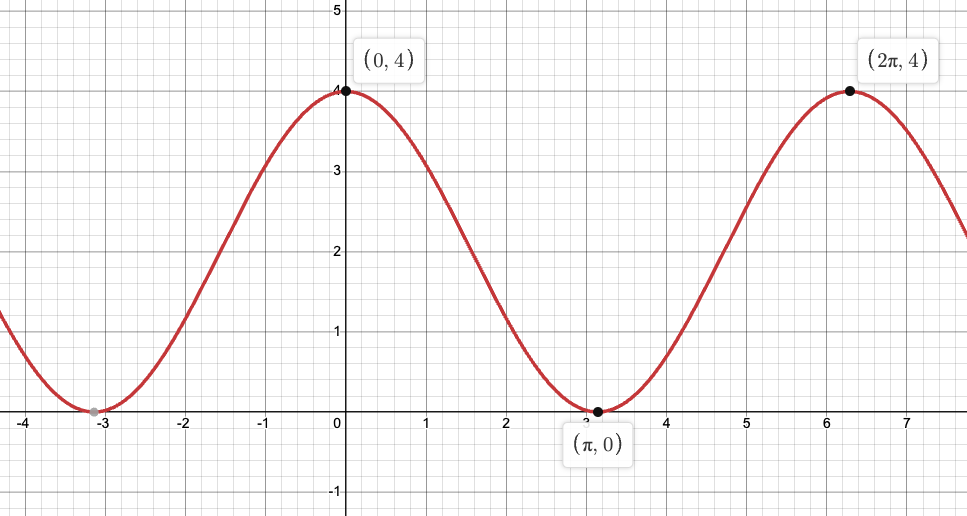
Answer: 2sin(x + (𝛑/2)) + 2
3.
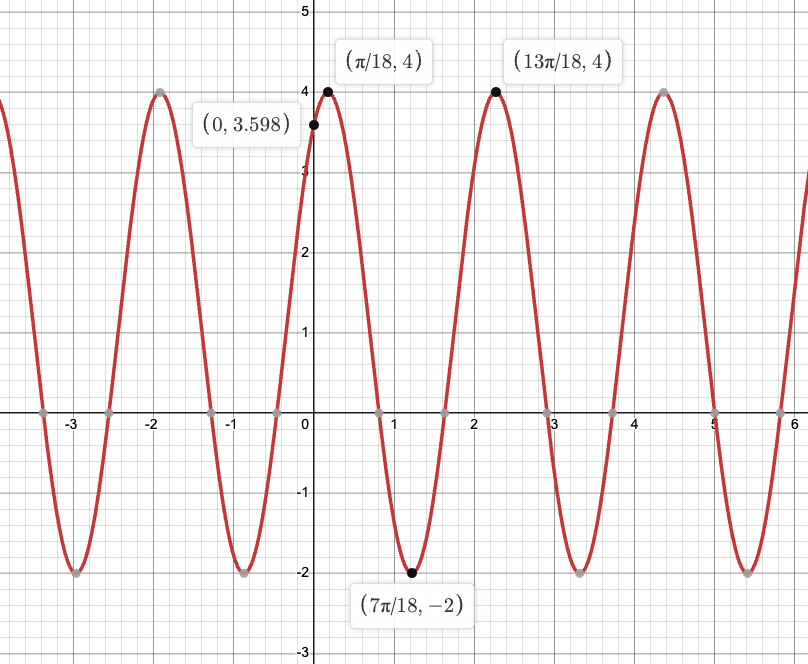
Answer: 3sin(3x + (𝛑/3)) + 1

© 2024 Fiveable Inc. All rights reserved.